Dynamics of melt spinning
processes accounting for the effects of flow-induced crystallizations has been
studied by mathematical modeling and computers simulations of high-speed melt
spinning [1-3] and pneumatic processes [4-8]. Axial profiles of melt spinning
processes (polymer velocity, temperature, tensile stress and crystallinity as dependent on the process and material
parameters are determined. Effects of crystallization on the dynamics of melt
spinning and rheological behavior is discussed [1]. Effects of on-line zone
heating during melt spinning of PET fibres was
studied [2]. Crystallinity-dependent polymer viscosity results in
limited take-up velocity and filament thickness and bifurcation leading to
three regions of the processing conditions leading to amorphous, partly
crystalline and inaccessible ranges [3].
A model for stationary melt blowing of
nonwovens [4-6] and pneumatic melt spinning by ultrasonic air jet in the Laval
nozzle [7,8] is proposed and applied for iPP and PLA
with the effects of on-line stress-induced crystallization on the polymer
viscosity and rheological relaxation time. The role of the viscous friction heat in the polymer bulk has
been discussed for fast air-drawing of the polymer melt. Axial profiles of the
polymer velocity, temperature elongation rate, filament diameter, tensile
stress, and extra-pressure were computed for the pneumatic processes of
non-woven formation. The effects of the air-jet velocity, die-to-collector
distance and polymer molecular weight are discussed.
The model allows to discuss non-linear stress-optical relationship reflecting
online molecular orientation, as well as online crystallization of the polymer
filament if it occurs. Significant online
extra-pressure in the filament was predicted in the case of supersonic air jets
as resulting from polymer viscoelasticity, which
could lead to longitudinal splitting into sub-filaments [5-7].
The filament velocity at the collector increases
significantly with increasing air compression, from the values typical for
high-speed melt spinning up to values by two folds higher. The increase in
filament velocity is limited by the effects of online oriented crystallization
at higher air compressions. Influence of the inlet air compression, melt
extrusion temperature and weight-average molecular weight on the axial profiles
of the melt spinning functions is discussed, as well as on the development of
amorphous orientation and online oriented crystallization.
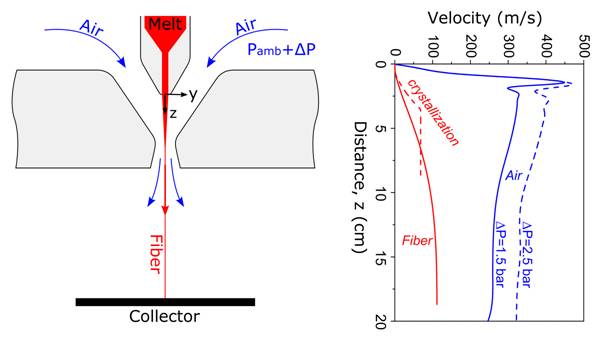
Cross-sections of the longitudinal die
assembly with the
[1]. Ziabicki A., Jarecki
L., Sorrentino A., The role of flow-induced crystallization in melt spinning, E-POLYMERS, no. 072, 2004.
[2]. Blim A., Jarecki
L., Effects of zone heating on PET fibers
structures and dynamics of melt spinning process.
Part II. Mathematical model),
POLIMERY, 52, 686-700, 2007.
[3]. Ziabicki A., Jarecki
L., Crystallization-controlled
limitations of melt spinning, JOURNAL OF APPLIED POLYMER SCIENCE, 105,
215-223, 2007.
[4]. Jarecki L., Ziabicki
A., Mathematical modeling of the
pneumatic melt spinning of isotactic polypropylene Part II. Dynamic model of melt blowing, FIBRES AND TEXTILES IN EASTERN EUROPE, 16,
17-24, 2008.
[5]. Jarecki L., Ziabicki A., Lewandowski Z., Dynamics
of air drawing in the melt blowing of nonwovens from isotactic polypropylene by computer
modeling, JOURNAL OF APPLIED POLYMER SCIENCE, 119, 53-65, 2011.
[6]. Jarecki L., Błoński S., Zachara A., Blim A., Computer
modeling of pneumatic formation of superthin fibres, COMPUTER METHODS IN MATERIALS SCIENCE /
INFORMATYKA W TECHNOLOGII MATERIAŁÓW, 11, 74-80, 2011.
[7]. Jarecki
L., Błoński S., Blim A., Zachara A., Modeling
of pneumatic melt spinning processes, JOURNAL OF APPLIED POLYMER SCIENCE, 125, 4402-4415, 2012.
[8]. Jarecki L., Błoński S., Zachara A., Modeling of
Pneumatic Melt Drawing of Poly-L-lactide
Fibers in the